Section G.4 – Circles
Circles are a common shape. You see them all over—wheels on a car, Frisbees passing through the air, DVD’s bringing you entertainment. These are all circles.
A circle is a two-dimensional figure just like polygons and quadrilaterals. However, circles are measured differently than these other shapes—you even have to use some different terms to describe them. Let’s take a look at this interesting shape.
Properties of Circles
A circle represents a set of points, all of which are the same distance away from a fixed, middle point. This fixed point is called the center. The distance from the center of the circle to any point on the circle is called the radius. When two radii (the plural of radius) are put together to form a line segment across the circle, you have a diameter. The diameter of a circle passes through the center of the circle and has its endpoints on the circle itself.
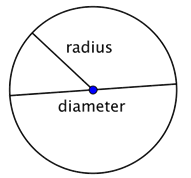
The diameter of any circle is two times the length of that circle’s radius. It can be represented by the expression 2r, or “two times the radius.” So if you know a circle’s radius, you can multiply it by 2 to find the diameter; this also means that if you know a circle’s diameter, you can divide by 2 to find the radius.
| Example 1 | |
|
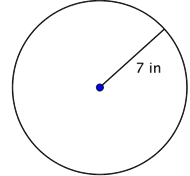
Find the diameter of the circle.
The diameter is two times the radius, or 2r. The radius of this circle is 7 inches, so the diameter is 2(7) = 14 inches. |
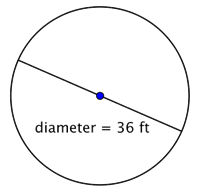
Find the radius of the circle.
The radius is half the diameter, or [latex]\frac{1}{2}[/latex]d. The diameter of this circle is 36 feet, so the radius is [latex]\frac{1}{2}[/latex](36) = 18 feet. |
Circumference
The distance around a circle is called the circumference. (Recall, the distance around a polygon is the perimeter.) One interesting property about circles is that the ratio of a circle’s circumference and its diameter is the same for all circles. No matter the size of the circle, the ratio of the circumference and diameter will be the same.
Some actual measurements of different items are provided below. The measurements are accurate to the nearest millimeter or quarter inch (depending on the unit of measurement used). Look at the ratio of the circumference to the diameter for each one—although the items are different, the ratio for each is approximately the same.
|
Item
|
Circumference (C) (rounded to nearest hundredth)
|
Diameter (d)
|
Ratio ([latex]\frac{C}{d}[/latex])
|
|
Cup |
253 mm |
79 mm |
[latex]\frac{253}{9}[/latex] = 3.2025… |
|
Quarter |
84 mm |
27 mm |
[latex]\frac{84}{27}[/latex] = 3.1111… |
|
Bowl |
37.25 in |
11.75 in |
[latex]\frac{37.25}{11.75}[/latex] = 3.1702… |
The circumference and the diameter are approximate measurements, since there is no precise way to measure these dimensions exactly. If you were able to measure them more precisely, however, you would find that the ratio [latex]\frac{C}{d}[/latex] approaches the value 3.14 for each of the items given. The mathematical name for this ratio is pi, and is represented by the Greek letter π.
Pi is a non-terminating, non-repeating decimal, so it is impossible to write it out completely. The first 10 digits of π are 3.141592653; it is often rounded to 3.14 or estimated as the fraction [latex]\frac{22}{7}[/latex]. Note that both 3.14 and [latex]\frac{22}{7}[/latex] are approximations of π, and are used in calculations where it is not important to be precise.
Since you know that the ratio of circumference to diameter (or π) is consistent for all circles, you can use this number to find the circumference of a circle if you know its diameter.
[latex]\frac{C}{d}[/latex] = π, so C = πd
Also, since d = 2r, then C = πd = π(2r) = 2πr.
|
Circumference of a Circle |
|
To find the circumference (C) of a circle, use one of the following formulas: If you know the diameter (d) of a circle: C = πd If you know the radius (r) of a circle: C = 2πr |
| Example 1 | ||
|
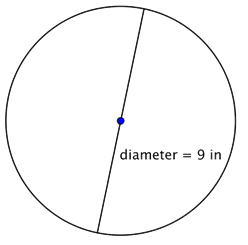
Find the circumference.
|
C = πd |
To calculate the circumference given a diameter of 9 inches, use the formula C = πd. Use 3.14 as an approximation for π. Since you are using an approximation for , you cannot give an exact measurement of the circumference. Instead, you use the symbol to indicate “approximately equal to.” |
|
The circumference is 9π, or approximately 28.26 inches. |
||
| Area of a Circle |
|
To find the area (A) of a circle, use the formula: A = πr2 Where r is the radius of the circle. |
| Example 2 | ||
|
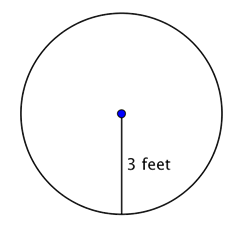
Find the area of the circle.
|
A = πr2
|
To find the area of this circle, use the formula A = πr2. Remember to write the answer in terms of square units, since you are finding the area. |
|
The area is 9π or approximately 28.26 feet2. Exact: 9π ft2 Approximate: 28.26 ft2 |
||
|
You Try G.4.A |
| A circle has a radius of 8 inches. Determine its area and circumference. Write your answers in exact form and in approximate form, rounded to the nearest tenth. Be sure to include correct units in your answers. |
Section G.4 – Answers to You Try Problems
G.4.A
The area is 64π or approximately 201.1 in2.
The circumference is 16π or approximately 50.3 inches.