4.7 – Attributes of Standard Deviations

Much like we discussed with the mean, it can be helpful to know some of the following attributes of the standard deviation:

• If you add a constant to or subtract a constant from every score in a distribution, the standard deviation does not change
• If you multiply every score in a distribution by a constant, you can multiply the standard deviation by that same constant

Adding or subtracting a score simply shifts scores higher or lower, but it doesn’t have any effect on the amount of spread in the scores. Remember in our discussion about the attributes of the mean we discussed the set of n = 4 scores: 2, 2, 6, 10

The mean, which is 5, is the balance point for those 4 scores because it balances out the deviations on either side of the mean:

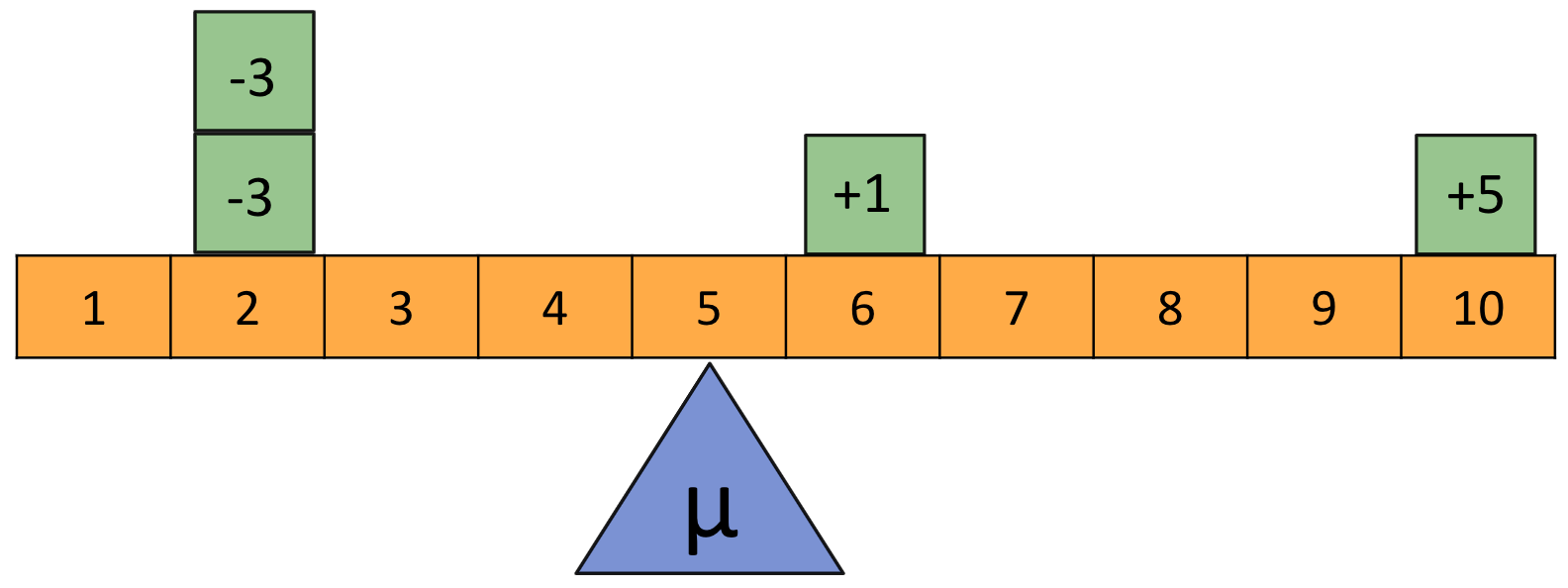
However, if we were to add a constant to each score, such as 10, we would get the following scores: 12, 12, 16, 20. Then, the mean also needs to shift up 10 points in order for things to balance, and thus the mean is now 5 + 10 = 15.

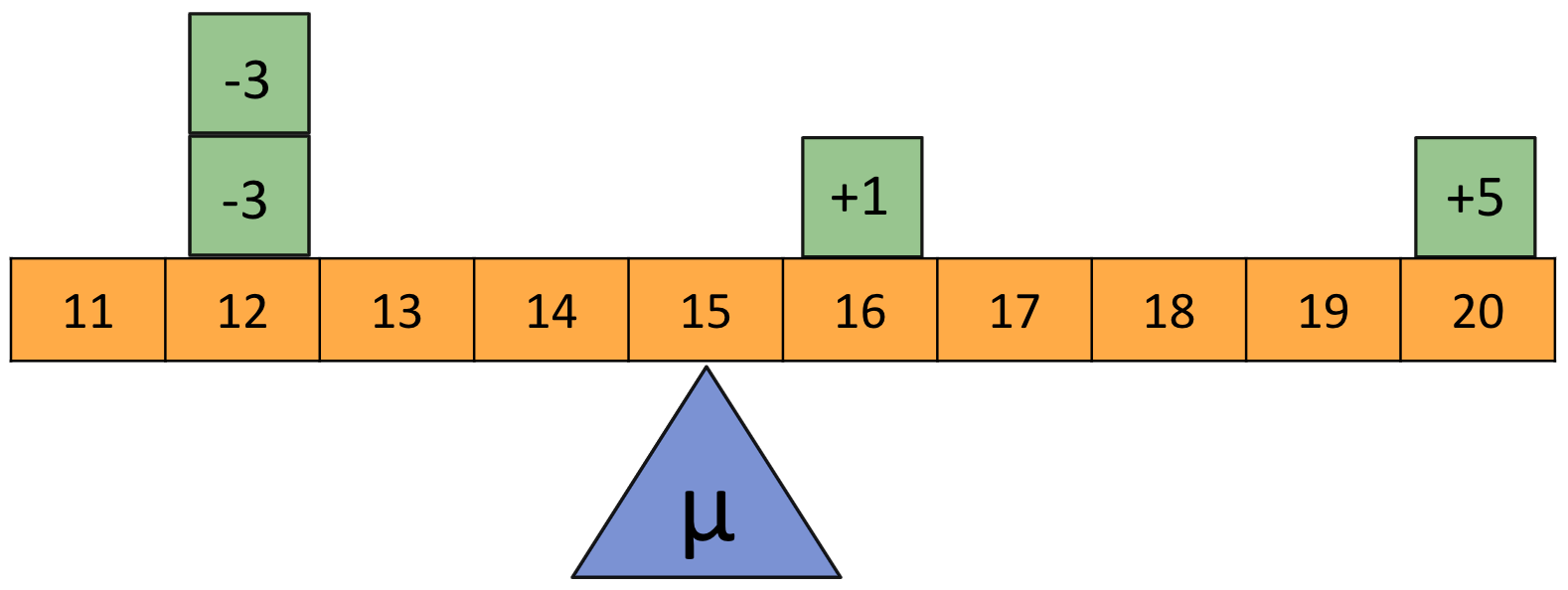
In contrast to the fact that the mean changes if we add a constant to each score, though, the standard deviation for these two sets of scores stays exactly the same. We can see that the deviations for each of the scores (the numbers inside of each green box) have not changed, and remember that the standard deviation measures the average deviation.  Thus, the standard deviation will remain the same even if you add or subtract a constant. In fact, the standard deviation for each set of scores is 3.317.

This should also make sense visually. Remember that standard deviation is a measure of spread, and looking at the green boxes that represent each of the four scores in each group, you can see that they are equally spread out. The spread hasn’t changed, and thus the standard deviation won’t either.

On the other hand, if you multiply all the scores in a distribution by a constant, then the standard deviation will change (it can be multiplied by that same constant).

Let’s take the original scores above: 2, 2, 6, and 10. If we multiply each of the scores by 2, we get the following scores: 4, 4, 12, 20. As we know from the characteristics of the mean, the mean for this new set of scores will equal the original mean multiplied by 2, which will be 5 x 2 = 10. In other words, the balance point for the four scores is 10:

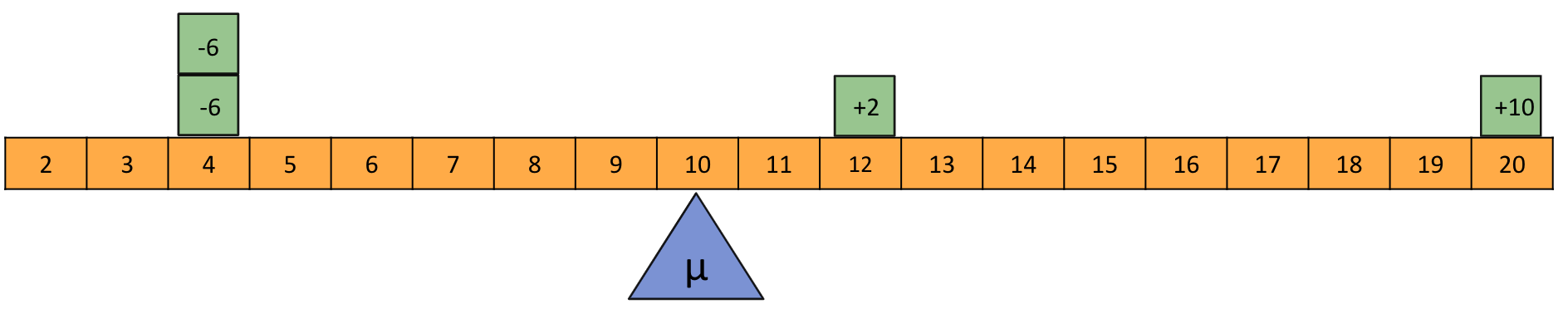
However, notice how the scores are now much more spread out than they were originally, and because the standard deviation measures the spread of the scores, the standard deviation should be larger than it was originally. In fact, we can just take the original standard deviation, which was 3.317, and multiply it by the same constant, 2, and we get the standard deviation for the new set of scores, 3.317 x 2 = 6.634. This indicates that the new set of scores has twice as much spread as the original set of scores.