1
The Common Core State Standards for Mathematics (http://www.thecorestandards.org/Math/Practice) identify eight “Mathematical Practices” — the kinds of expertise that all teachers should try to foster in their students, but they go far beyond any particular piece of mathematics content. They describe what mathematics is really about, and why it is so valuable for students to master. The very first Mathematical Practice is:
Make sense of problems and persevere in solving them. Mathematically proficient students start by explaining to themselves the meaning of a problem and looking for entry points to its solution. They analyze givens, constraints, relationships, and goals. They make conjectures about the form and meaning of the solution and plan a solution pathway rather than simply jumping into a solution attempt. They consider analogous problems, and try special cases and simpler forms of the original problem in order to gain insight into its solution. They monitor and evaluate their progress and change course if necessary.
This chapter will help you develop these very important mathematical skills, so that you will be better prepared to help your future students develop them. Let’s start with solving a problem!
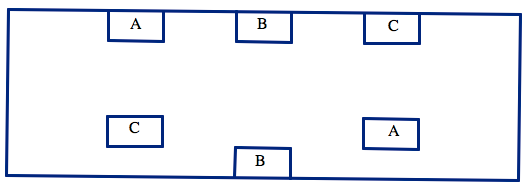
Problem 1 (ABC)
Draw curves connecting A to A, B to B, and C to C. Your curves cannot cross or even touch each other, they cannot cross through any of the lettered boxes, and they cannot go outside the large box or even touch it’s sides.
Think / Pair / Share
After you have worked on the problem on your own for a while, talk through your ideas with a partner (even if you have not solved it).
- What did you try?
- What makes this problem difficult?
- Can you change the problem slightly so that it would be easier to solve?
Problem Solving Strategy 1 (Wishful Thinking). Do you wish something in the problem was different? Would it then be easier to solve the problem?
For example, what if ABC problem had a picture like this:
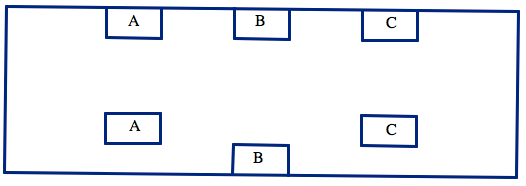
Can you solve this case and use it to help you solve the original case? Think about moving the boxes around once the lines are already drawn.
Here is one possible solution.

