Chapter 19: Nuclear Chemistry

Learning Outcomes

  • Recognize common modes of radioactive decay
  • Identify common particles and energies involved in nuclear decay reactions
  • Write and balance nuclear decay equations
  • Calculate kinetic parameters for decay processes, including half-life
  • Describe common radiometric dating techniques

Following the somewhat serendipitous discovery of radioactivity by Becquerel, many prominent scientists began to investigate this new, intriguing phenomenon. Among them were Marie Curie (the first woman to win a Nobel Prize, and the only person to win two Nobel Prizes in different sciences—chemistry and physics), who was the first to coin the term “radioactivity,” and Ernest Rutherford (of gold foil experiment fame), who investigated and named three of the most common types of radiation. During the beginning of the twentieth century, many radioactive substances were discovered, the properties of radiation were investigated and quantified, and a solid understanding of radiation and nuclear decay was developed.

The spontaneous change of an unstable nuclide into another is radioactive decay. The unstable nuclide is called the parent nuclide; the nuclide that results from the decay is known as the daughter nuclide. The daughter nuclide may be stable, or it may decay itself. The radiation produced during radioactive decay is such that the daughter nuclide lies closer to the band of stability than the parent nuclide, so the location of a nuclide relative to the band of stability can serve as a guide to the kind of decay it will undergo.

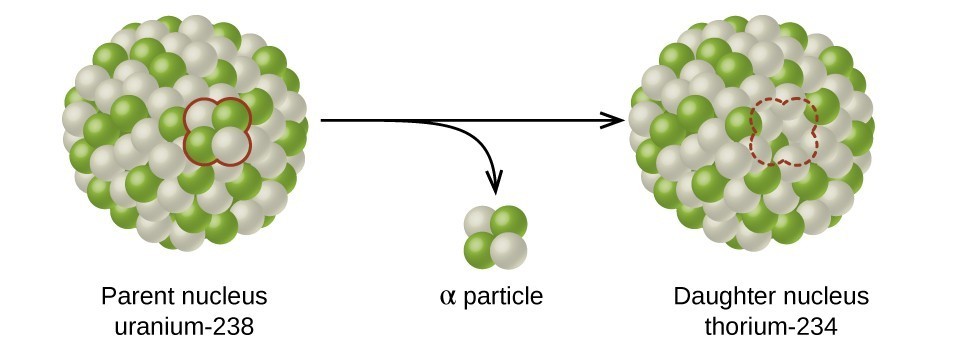
A diagram shows two spheres composed of many smaller white and green spheres connected by a right-facing arrow with another, down-facing arrow coming off of it. The left sphere, labeled “Parent nucleus uranium dash 238” has two white and two green spheres that are near one another and are outlined in red. These two green and two white spheres are shown near the tip of the down-facing arrow and labeled “alpha particle.” The right sphere, labeled “Daughter nucleus radon dash 234,” looks the same as the left, but has a space for four smaller spheres outlined with a red dotted line.
Figure 19.3.1. A nucleus of uranium-238 (the parent nuclide) undergoes α decay to form thorium-234 (the daughter nuclide). The alpha particle removes two protons (green) and two neutrons (gray) from the uranium-238 nucleus.

Types of Radioactive Decay

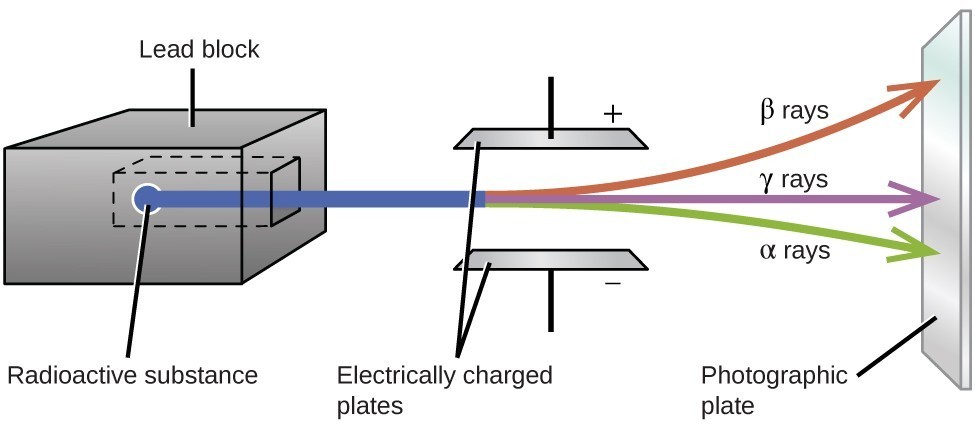
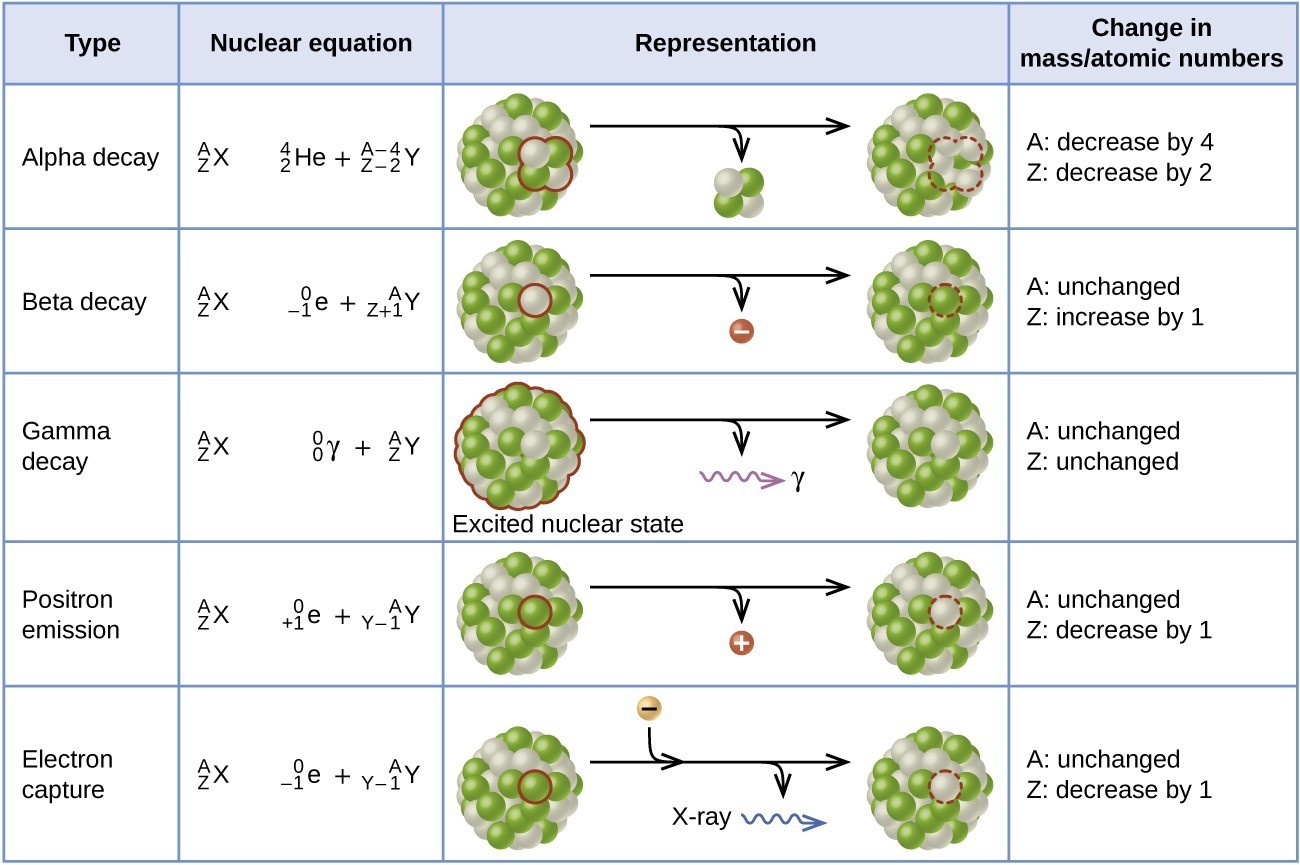
Ernest Rutherford’s experiments involving the interaction of radiation with a magnetic or electric field helped him determine that one type of radiation consisted of positively charged and relatively massive α particles; a second type was made up of negatively charged and much less massive β particles; and a third was uncharged electromagnetic waves, γ rays. We now know that α particles are high-energy helium nuclei, β particles are high-energy electrons, and γ radiation compose high-energy electromagnetic radiation. We classify different types of radioactive decay by the radiation produced.

A diagram is shown. A gray box on the left side of the diagram labeled “Lead block” has a chamber hollowed out in the center in which a sample labeled “Radioactive substance” is placed. A blue beam is coming from the sample, out of the block, and passing through two horizontally placed plates that are labeled “Electrically charged plates.” The top plate is labeled with a positive sign while the bottom plate is labeled with a negative sign. The beam is shown to break into three beams as it passes in between the plates; in order from top to bottom, they are red, labeled “beta rays,” purple labeled “gamma rays” and green labeled “alpha rays.” The beams are shown to hit a vertical plate labeled “Photographic plate” on the far right side of the diagram.
Figure 19.3.2. Alpha particles, which are attracted to the negative plate and deflected by a relatively small amount, must be positively charged and relatively massive. Beta particles, which are attracted to the positive plate and deflected a relatively large amount, must be negatively charged and relatively light. Gamma rays, which are unaffected by the electric field, must be uncharged.

Alpha (α) decay is the emission of an α particle from the nucleus. For example, polonium-210 undergoes α decay:

[latex]\ce{_{84}^{210}Po}\longrightarrow \ce{_2^4He}+\ce{_{82}^{206}Pb}\,\,\,\,\,\,\,\text{or}\,\,\,\,\,\,\,\ce{_{84}^{210}Po}\longrightarrow {}_{2}^{4}\alpha+\ce{_{82}^{206}Pb}[/latex]

Alpha decay occurs primarily in heavy nuclei (A > 200, Z > 83). Because the loss of an α particle gives a daughter nuclide with a mass number four units smaller and an atomic number two units smaller than those of the parent nuclide, the daughter nuclide has a larger n:p ratio than the parent nuclide. If the parent nuclide undergoing α decay lies below the band of stability, the daughter nuclide will lie closer to the band.

Beta (β) decay is the emission of an electron from a nucleus. Iodine-131 is an example of a nuclide that undergoes β decay:

[latex]\ce{_{53}^{131}I}\longrightarrow {}_{-1}^{0}\text{e}+{}_{54}^{131}\text{X}\,\,\,\,\,\,\,\text{or}\,\,\,\,\,\,\,\ce{_{53}^{131}I}\longrightarrow {}_{-1}^{0}\beta_{\phantom{}}^{\phantom{}}+\ce{_{54}^{131}Xe}[/latex]

Beta decay, which can be thought of as the conversion of a neutron into a proton and a β particle, is observed in nuclides with a large n:p ratio. The beta particle (electron) emitted is from the atomic nucleus and is not one of the electrons surrounding the nucleus. Such nuclei lie above the band of stability. Emission of an electron does not change the mass number of the nuclide but does increase the number of its protons and decrease the number of its neutrons. Consequently, the n:p ratio is decreased, and the daughter nuclide lies closer to the band of stability than did the parent nuclide.

Gamma emission (γ emission) is observed when a nuclide is formed in an excited state and then decays to its ground state with the emission of a γ ray, a quantum of high-energy electromagnetic radiation. The presence of a nucleus in an excited state is often indicated by an asterisk (*). Cobalt-60 emits γ radiation and is used in many applications including cancer treatment:

[latex]\ce{_{27}^{60}Co*}\longrightarrow {}_{0}^{0}\gamma+\ce{_{27}^{60}Co}[/latex]

There is no change in mass number or atomic number during the emission of a γ ray unless the γ emission accompanies one of the other modes of decay.

Positron emission (β+ decay) is the emission of a positron from the nucleus. Oxygen-15 is an example of a nuclide that undergoes positron emission:

[latex]\ce{_8^{15}O}\longrightarrow {}_{+1}^{0}\text{e}+\ce{_7^{15}N}\,\,\,\,\,\,\,\text{or}\,\,\,\,\,\,\,\ce{_{8}^{15}O}\longrightarrow {}_{+1}^{0}\beta_{\phantom{}}^{\phantom{}}+\ce{_7^{15}N}[/latex]

Positron emission is observed for nuclides in which the n:p ratio is low. These nuclides lie below the band of stability. Positron decay is the conversion of a proton into a neutron with the emission of a positron. The n:p ratio increases, and the daughter nuclide lies closer to the band of stability than did the parent nuclide.

Electron capture occurs when one of the inner electrons in an atom is captured by the atom’s nucleus. For example, potassium-40 undergoes electron capture:

[latex]\ce{_{19}^{40}K}+{}_{-1}^{0}\text{e}\longrightarrow \ce{_{18}^{40}Ar}[/latex]

Electron capture occurs when an inner shell electron combines with a proton and is converted into a neutron. The loss of an inner shell electron leaves a vacancy that will be filled by one of the outer electrons. As the outer electron drops into the vacancy, it will emit energy. In most cases, the energy emitted will be in the form of an X-ray. Like positron emission, electron capture occurs for “proton-rich” nuclei that lie below the band of stability. Electron capture has the same effect on the nucleus as does positron emission: The atomic number is decreased by one and the mass number does not change. This increases the n:p ratio, and the daughter nuclide lies closer to the band of stability than did the parent nuclide. Whether electron capture or positron emission occurs is difficult to predict. The choice is primarily due to kinetic factors, with the one requiring the smaller activation energy being the one more likely to occur.

Figure 19.3.3 summarizes these types of decay, along with their equations and changes in atomic and mass numbers.

This table has four columns and six rows. The first row is a header row and it labels each column: “Type,” “Nuclear equation,” “Representation,” and “Change in mass / atomic numbers.” Under the “Type” column are the following: “Alpha decay,” “Beta decay,” “Gamma decay,” “Positron emission,” and “Electron capture.” Under the “Nuclear equation” column are several equations. Each begins with superscript A stacked over subscript Z X. There is a large gap of space and then the following equations: “superscript 4 stacked over subscript 2 He plus superscript A minus 4 stacked over subscript Z minus 2 Y,” “superscript 0 stacked over subscript negative 1 e plus superscript A stacked over subscript Z plus 1 Y,” “superscript 0 stacked over subscript 0 lowercase gamma plus superscript A stacked over subscript Z Y,” “superscript 0 stacked over subscript positive 1 e plus superscript A stacked over subscript Y minus 1 Y,” and “superscript 0 stacked over subscript negative 1 e plus superscript A stacked over subscript Y minus 1 Y.” Under the “Representation” column are the five diagrams. The first shows a cluster of green and white spheres. A section of the cluster containing two white and two green spheres is outlined. There is a right-facing arrow pointing to a similar cluster as previously described, but the outlined section is missing. From the arrow another arrow branches off and points downward. The small cluster to two white spheres and two green spheres appear at the end of the arrow. The next diagram shows the same cluster of white and green spheres. One white sphere is outlined. There is a right-facing arrow to a similar cluster, but the white sphere is missing. Another arrow branches off the main arrow and a red sphere with a negative sign appears at the end. The next diagram shows the same cluster of white and green spheres. The whole sphere is outlined and labeled, “excited nuclear state.” There is a right-facing arrow that points to the same cluster. No spheres are missing. Off the main arrow is another arrow which points to a purple squiggle arrow which in turn points to a lowercase gamma. The next diagram shows the same cluster of white and green spheres. One green sphere is outlined. There is a right-facing arrow to a similar cluster, but the green sphere is missing. Another arrow branches off the main arrow and a red sphere with a positive sign appears at the end. The next diagram shows the same cluster of white and green spheres. One green sphere is outlined. There is a right-facing arrow to a similar cluster, but the green sphere is missing. Two other arrows branch off the main arrow. The first shows a gold sphere with a negative sign joining with the right-facing arrow. The secon points to a blue squiggle arrow labeled, “X-ray.” Under the “Change in mass / atomic numbers” column are the following: “A: decrease by 4, Z: decrease by 2,” “A: unchanged, Z: increased by 1,” “A: unchanged, Z: unchanged,” “A: unchanged, Z: unchanged,” “A: unchanged, Z: decrease by 1,” and “A: unchanged, Z: decrease by 1.”
Figure 19.3.3. This table summarizes the type, nuclear equation, representation, and any changes in the mass or atomic numbers for various types of decay.

PET Scan

Positron emission tomography (PET) scans use radiation to diagnose and track health conditions and monitor medical treatments by revealing how parts of a patient’s body function (Figure 19.3.4). To perform a PET scan, a positron-emitting radioisotope is produced in a cyclotron and then attached to a substance that is used by the part of the body being investigated. This “tagged” compound, or radiotracer, is then put into the patient (injected via IV or breathed in as a gas), and how it is used by the tissue reveals how that organ or other area of the body functions.

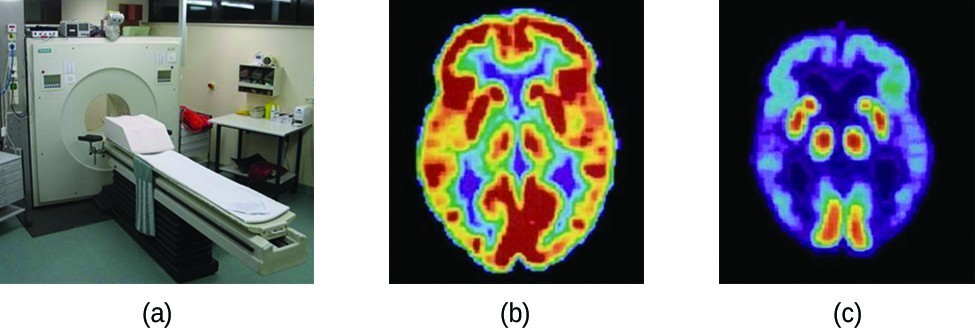
Three pictures are shown and labeled “a,” “b” and “c.” Picture a shows a machine with a round opening connected to an examination table. Picture b is a medical scan of the top of a person’s head and shows large patches of yellow and red and smaller patches of blue, green and purple highlighting. Picture c also shows a medical scan of the top of a person’s head, but this image is mostly colored in blue and purple with very small patches of red and yellow.
Figure 19.3.4. A PET scanner (a) uses radiation to provide an image of how part of a patient’s body functions. The scans it produces can be used to image a healthy brain (b) or can be used for diagnosing medical conditions such as Alzheimer’s disease (c). (credit a: modification of work by Jens Maus)

For example, F-18 is produced by proton bombardment of [latex]\ce{^{18}O}[/latex] ([latex]\ce{_8^{18}O}+{}_{1}^{1}\text{p}\longrightarrow \ce{_9^{18}F}+{}_{0}^{1}\text{n}[/latex]) and incorporated into a glucose analog called fludeoxyglucose (FDG). How FDG is used by the body provides critical diagnostic information; for example, since cancers use glucose differently than normal tissues, FDG can reveal cancers. The 18F emits positrons that interact with nearby electrons, producing a burst of gamma radiation. This energy is detected by the scanner and converted into a detailed, three-dimensional, color image that shows how that part of the patient’s body functions. Different levels of gamma radiation produce different amounts of brightness and colors in the image, which can then be interpreted by a radiologist to reveal what is going on. PET scans can detect heart damage and heart disease, help diagnose Alzheimer’s disease, indicate the part of a brain that is affected by epilepsy, reveal cancer, show what stage it is, and how much it has spread, and whether treatments are effective. Unlike magnetic resonance imaging and X-rays, which only show how something looks, the big advantage of PET scans is that they show how something functions. PET scans are now usually performed in conjunction with a computed tomography scan.

Radioactive Decay Series

The naturally occurring radioactive isotopes of the heaviest elements fall into chains of successive disintegrations, or decays, and all the species in one chain constitute a radioactive family, or radioactive decay series. Three of these series include most of the naturally radioactive elements of the periodic table. They are the uranium series, the actinide series, and the thorium series. The neptunium series is a fourth series, which is no longer significant on the earth because of the short half-lives of the species involved. Each series is characterized by a parent (first member) that has a long half-life and a series of daughter nuclides that ultimately lead to a stable end-product—that is, a nuclide on the band of stability (Figure 19.3.5). In all three series, the end-product is a stable isotope of lead. The neptunium series, previously thought to terminate with bismuth-209, terminates with thallium-205.

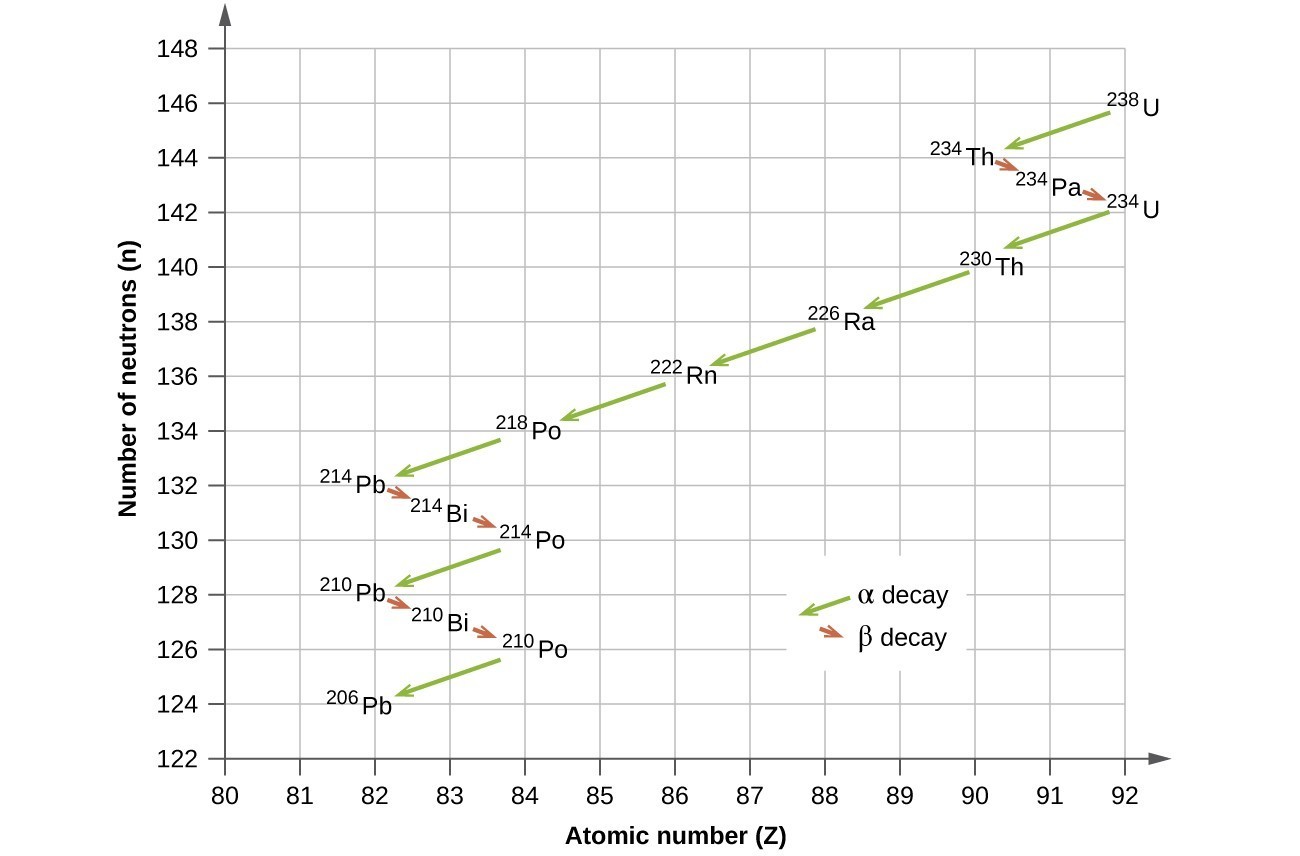
A graph is shown where the x-axis is labeled “Number of neutrons, open parenthesis, n, close parenthesis” and has values of 122 to 148 in increments of 2. The y-axis is labeled “Atomic number” and has values of 80 to 92 in increments of 1. Two types of arrows are used in this graph to connect the points. Green arrows are labeled as “alpha decay” while red arrows are labeled “beta decay.” Beginning at the point “92, 146” that is labeled “superscript 238, U,” a green arrow connects this point to the second point “90, 144” which is labeled “superscript 234, T h.” A red arrow connect this to the third point “91, 143” which is labeled “superscript 234, P a” which is connected to the fourth point “92, 142” by a red arrow and which is labeled “superscript 234, U.” A green arrow leads to the next point, “90, 140” which is labeled “superscript 230, T h” and is connected by a green arrow to the sixth point, “88, 138” which is labeled “superscript 226, R a” that is in turn connected by a green arrow to the seventh point “86, 136” which is labeled “superscript 222, Ra.” The eighth point, at “84, 134” is labeled “superscript 218, P o” and has green arrows leading to it and away from it to the ninth point “82, 132” which is labeled “superscript 214, Pb” which is connected by a red arrow to the tenth point, “83, 131” which is labeled “superscript 214, B i.” A red arrow leads to the eleventh point “84, 130” which is labeled “superscript 214, P o” and a green arrow leads to the twelvth point “82, 128” which is labeled “superscript 210, P b.” A red arrow leads to the thirteenth point “83, 127” which is labeled “superscript 210, B i” and a red arrow leads to the fourteenth point “84, 126” which is labeled “superscript 210, P o.” The final point is labeled “82, 124” and “superscript 206, P b.”
Figure 19.3.5. Uranium-238 undergoes a radioactive decay series consisting of 14 separate steps before producing stable lead-206. This series consists of eight α decays and six β decays.

Radioactive Half-Lives

Radioactive decay follows first-order kinetics. Since first-order reactions have already been covered in detail in the kinetics chapter , we will now apply those concepts to nuclear decay reactions. Each radioactive nuclide has a characteristic, constant half-life (t1/2), the time required for half of the atoms in a sample to decay. An isotope’s half-life allows us to determine how long a sample of a useful isotope will be available, and how long a sample of an undesirable or dangerous isotope must be stored before it decays to a low-enough radiation level that is no longer a problem.

For example, cobalt-60, an isotope that emits gamma rays used to treat cancer, has a half-life of 5.27 years (Figure 19.3.6). In a given cobalt-60 source, since half of the [latex]\ce{_{27}^{60}Co}[/latex] nuclei decay every 5.27 years, both the amount of material and the intensity of the radiation emitted is cut in half every 5.27 years. (Note that for a given substance, the intensity of radiation that it produces is directly proportional to the rate of decay of the substance and the amount of the substance.) This is as expected for a process following first-order kinetics. Thus, a cobalt-60 source that is used for cancer treatment must be replaced regularly to continue to be effective.

A graph, titled “C o dash 60 Decay,” is shown where the x-axis is labeled “C o dash 60 remaining, open parenthesis, percent sign, close parenthesis” and has values of 0 to 100 in increments of 25. The y-axis is labeled “Number of half dash lives” and has values of 0 to 5 in increments of 1. The first point, at “0, 100” has a circle filled with tiny dots drawn near it labeled “10 g.” The second point, at “1, 50” has a smaller circle filled with tiny dots drawn near it labeled “5 g.” The third point, at “2, 25” has a small circle filled with tiny dots drawn near it labeled “2.5 g.” The fourth point, at “3, 12.5” has a very small circle filled with tiny dots drawn near it labeled “1.25 g.” The last point, at “4, 6.35” has a tiny circle filled with tiny dots drawn near it labeled.”625 g.”
Figure 19.3.6. For cobalt-60, which has a half-life of 5.27 years, 50% remains after 5.27 years (one half-life), 25% remains after 10.54 years (two half-lives), 12.5% remains after 15.81 years (three half-lives), and so on.

Since nuclear decay follows first-order kinetics, we can adapt the mathematical relationships used for first-order chemical reactions. We generally substitute the number of nuclei, N, for the concentration. If the rate is stated in nuclear decays per second, we refer to it as the activity of the radioactive sample. The rate for radioactive decay is:

decay rate = λN with λ = the decay constant for the particular radioisotope

The decay constant, λ, which is the same as a rate constant discussed in the kinetics chapter. It is possible to express the decay constant in terms of the half-life, t1/2:

[latex]\lambda =\dfrac{\text{ln 2}}{{t}_{1\text{/}2}}=\dfrac{0.693}{{t}_{1\text{/}2}}\,\,\,\,\,\,\,\text{or}\,\,\,\,\,\,\,{t}_{1\text{/}2}=\dfrac{\text{ln 2}}{\lambda }=\dfrac{0.693}{\lambda }[/latex]

The first-order equations relating amount, N, and time are:

[latex]{N}_{t}={N}_{0}{e}^{-kt}\,\,\,\,\,\,\,\text{or}\,\,\,\,\,\,\,t=-\dfrac{1}{\lambda }\text{ln}\left(\dfrac{{N}_{t}}{{N}_{0}}\right)[/latex]

where N0 is the initial number of nuclei or moles of the isotope, and Nt is the number of nuclei/moles remaining at time t. Example 19.3.1 applies these calculations to find the rates of radioactive decay for specific nuclides.

Example 19.3.1: Rates of Radioactive Decay

[latex]\ce{_{27}^{60}Co}[/latex] decays with a half-life of 5.27 years to produce [latex]\ce{_{28}^{60}Ni}[/latex].

  1. What is the decay constant for the radioactive disintegration of cobalt-60?
  2. Calculate the fraction of a sample of the [latex]\ce{_{27}^{60}Co}[/latex] isotope that will remain after 15 years.
  3. How long does it take for a sample of [latex]\ce{_{27}^{60}Co}[/latex] to disintegrate to the extent that only 2.0% of the original amount remains?

[reveal-answer q=”569746″]Show Solution[/reveal-answer]
[hidden-answer a=”569746″]

  1. The value of the rate constant is given by:[latex]\lambda =\dfrac{\text{ln 2}}{{t}_{1\text{/}2}}=\dfrac{0.693}{5.27\text{y}}=0.132{\text{y}}^{-1}[/latex]
  2. The fraction of [latex]\ce{_{27}^{60}Co}[/latex] that is left after time t is given by [latex]\dfrac{{N}_{t}}{{N}_{0}}[/latex]. Rearranging the first-order relationship Nt = N0e–λt to solve for this ratio yields:[latex]\dfrac{{N}_{t}}{{N}_{0}}={e}^{-\lambda t}={e}^{-\left(0.132\text{/y}\right)\left(15.0(y)\right)}=0.138[/latex]The fraction of [latex]\ce{_{27}^{60}Co}[/latex] that will remain after 15.0 years is 0.138. Or put another way, 13.8% of the [latex]\ce{_{27}^{60}Co}[/latex] originally present will remain after 15 years.
  3. 2.00% of the original amount of [latex]\ce{_{27}^{60}Co}[/latex] is equal to 0.0200 [latex]\times[/latex] N0. Substituting this into the equation for time for first-order kinetics, we have:

[latex]t=-\dfrac{1}{\lambda }\text{ln}\left(\dfrac{{N}_{t}}{{N}_{0}}\right)=-\dfrac{1}{0.132{\text{y}}^{-1}}\text{ln}\left(\dfrac{0.0200\times {N}_{0}}{{N}_{0}}\right)=29.6\text{y}[/latex]

[/hidden-answer]

Check Your Learning

Because each nuclide has a specific number of nucleons, a particular balance of repulsion and attraction, and its own degree of stability, the half-lives of radioactive nuclides vary widely. For example: the half-life of [latex]\ce{_{83}^{209}Bi}[/latex] is 1.9 [latex]\times[/latex] 1019 years; [latex]\ce{_{94}^{239}Ra}[/latex] is 24,000 years; [latex]\ce{_{86}^{222}Rn}[/latex] is 3.82 days; and element-111 (Rg for roentgenium) is 1.5 [latex]\times[/latex] 10–3 seconds. The half-lives of a number of radioactive isotopes important to medicine are shown in Table 19.3.1, and others are listed in Half-Lives for Several Radioactive Isotopes.

 

 

Table 19.3.1. Half-lives of Radioactive Isotopes Important to Medicine
Type[1] Decay Mode Half-Life Uses
F-18 β+ decay 110. minutes PET scans
Co-60 β decay, γ decay 5.27 years cancer treatment
Tc-99m γ decay 8.01 hours scans of brain, lung, heart, bone
I-131 β decay 8.02 days thyroid scans and treatment
Tl-201 electron capture 73 hours heart and arteries scans; cardiac stress tests

Radiometric Dating

Several radioisotopes have half-lives and other properties that make them useful for purposes of “dating” the origin of objects such as archaeological artifacts, formerly living organisms, or geological formations. This process is radiometric dating and has been responsible for many breakthrough scientific discoveries about the geological history of the earth, the evolution of life, and the history of human civilization. We will explore some of the most common types of radioactive dating and how the particular isotopes work for each type.

Radioactive Dating Using Carbon-14

The radioactivity of carbon-14 provides a method for dating objects that were a part of a living organism. This method of radiometric dating, which is also called radiocarbon dating or carbon-14 dating, is accurate for dating carbon-containing substances that are up to about 30,000 years old, and can provide reasonably accurate dates up to a maximum of about 50,000 years old.

Naturally occurring carbon consists of three isotopes: [latex]\ce{_6^{12}C}[/latex], which constitutes about 99% of the carbon on earth; [latex]\ce{_6^{13}C}[/latex], about 1% of the total; and trace amounts of [latex]\ce{_6^{14}C}[/latex]. Carbon-14 forms in the upper atmosphere by the reaction of nitrogen atoms with neutrons from cosmic rays in space:

[latex]\ce{_7^{14}N}+{}_{0}^{1}\text{n}\longrightarrow \ce{_6^{14}C}+\ce{_1^1H}[/latex]

All isotopes of carbon react with oxygen to produce [latex]\ce{CO2}[/latex] molecules. The ratio of [latex]\ce{_6^{14}CO2}[/latex] to [latex]\ce{_6^{12}CO2}[/latex] depends on the ratio of [latex]\ce{_6^{14}CO}[/latex] to [latex]\ce{_6^{12}CO}[/latex] in the atmosphere. The natural abundance of [latex]\ce{_6^{14}CO}[/latex] in the atmosphere is approximately 1 part per trillion; until recently, this has generally been constant over time, as seen is gas samples found trapped in ice. The incorporation of [latex]\ce{_6^{14}CO2}[/latex] and [latex]\ce{_6^{12}CO2}[/latex] into plants is a regular part of the photosynthesis process, which means that the [latex]\ce{_6^{14}C:_6^{12}C}[/latex] ratio found in a living plant is the same as the [latex]\ce{_6^{14}C:_6^{12}C}[/latex] ratio in the atmosphere. But when the plant dies, it no longer traps carbon through photosynthesis. Because [latex]\ce{_6^{12}C}[/latex] is a stable isotope and does not undergo radioactive decay, its concentration in the plant does not change. However, carbon-14 decays by β emission with a half-life of 5730 years:

[latex]\ce{_6^{14}C}\longrightarrow \ce{_7^{14}N}+{}_{-1}^{0}\text{e}[/latex]

Thus, the [latex]\ce{_6^{14}C:_6^{12}C}[/latex] ratio gradually decreases after the plant dies. The decrease in the ratio with time provides a measure of the time that has elapsed since the death of the plant (or other organism that ate the plant). Figure 19.3.7 visually depicts this process.

A diagram shows a cow standing on the ground next to a tree. In the upper left of the diagram, where the sky is represented, a single white sphere is shown and is connected by a downward-facing arrow to a larger sphere composed of green and white spheres that is labeled “superscript 14, subscript 7, N.” This structure is connected to three other structures by a right-facing arrow. Each of the three it points to are composed of green and white spheres and all have arrows pointing from them to the ground. The first of these is labeled “Trace, superscript 14, subscript 6, C,” the second is labeled “1 percent, superscript 13, subscript 6, C” and the last is labeled “99 percent, superscript 12, subscript 6, C.” Two downward-facing arrows that merge into one arrow lead from the cow and tree to the ground and are labeled “organism dies” and “superscript 14, subscript 6, C, decay begins.” A right-facing arrow labeled on top as “Decay” and on bottom as “Time” leads from this to a label of “superscript 14, subscript 6, C, backslash, superscript 12, subscript 6, C, ratio decreased.” Near the top of the tree is a downward facing arrow with the label “superscript 14, subscript 6, C, backslash, superscript 12, subscript 6, C, ratio is constant in living organisms” that leads to the last of the lower statements.
Figure 19.3.7. Along with stable carbon-12, radioactive carbon-14 is taken in by plants and animals, and remains at a constant level within them while they are alive. After death, the [latex]\ce{C{-}14}[/latex] decays and the [latex]\ce{C{-}14:C{-}12}[/latex] ratio in the remains decreases. Comparing this ratio to the [latex]\ce{C{-}14:C{-}12}[/latex] ratio in living organisms allows us to determine how long ago the organism lived (and died).

For example, with the half-life of [latex]\ce{_6^{14}C}[/latex] being 5730 years, if the [latex]\ce{_6^{14}C:_6^{12}C}[/latex] ratio in a wooden object found in an archaeological dig is half what it is in a living tree, this indicates that the wooden object is 5730 years old. Highly accurate determinations of [latex]\ce{_6^{14}C:_6^{12}C}[/latex] ratios can be obtained from very small samples (as little as a milligram) by the use of a mass spectrometer.

Example 19.3.2: Radiocarbon Dating

A tiny piece of paper (produced from formerly living plant matter) taken from the Dead Sea Scrolls has an activity of 10.8 disintegrations per minute per gram of carbon. If the initial [latex]\ce{C{-}14}[/latex] activity was 13.6 disintegrations/min/g of [latex]\ce{C}[/latex], estimate the age of the Dead Sea Scrolls.
[reveal-answer q=”788103″]Show Solution[/reveal-answer]
[hidden-answer a=”788103″]

The rate of decay (number of disintegrations/minute/gram of carbon) is proportional to the amount of radioactive [latex]\ce{C{-}14}[/latex] left in the paper, so we can substitute the rates for the amounts, N, in the relationship:

[latex]t=-\dfrac{1}{\lambda }\text{ln}\left(\dfrac{{N}_{t}}{{N}_{0}}\right)\longrightarrow t=-\dfrac{1}{\lambda }\text{ln}\left(\dfrac{{\text{Rate}}_{t}}{{\text{Rate}}_{0}}\right)[/latex]

where the subscript 0 represents the time when the plants were cut to make the paper, and the subscript t represents the current time.

The decay constant can be determined from the half-life of [latex]\ce{C{-}14}[/latex], 5730 years:

[latex]\lambda =\dfrac{\text{ln 2}}{{t}_{1\text{/}2}}=\dfrac{0.693}{\text{5730 y}}=1.21\times {10}^{-4}{\text{y}}^{-1}[/latex].

Substituting and solving, we have:

[latex]t=-\dfrac{1}{\lambda }\text{ln}\left(\dfrac{{\text{Rate}}_{t}}{{\text{Rate}}_{0}}\right)=-\dfrac{1}{1.21\times {10}^{-4}{\text{y}}^{-1}}\text{ln}\left(\dfrac{10.8\text{dis/min/g C}}{13.6\text{dis/min/g C}}\right)=\text{1910 y}[/latex].

Therefore, the Dead Sea Scrolls are approximately 1900 years old (Figure 19.3.8).

A photograph of six pages of ragged-edged paper covered in writing are shown.
Figure 19.3.8. Carbon-14 dating has shown that these pages from the Dead Sea Scrolls were written or copied on paper made from plants that died between 100 BCE and CE 50.

[/hidden-answer]

Check Your Learning

There have been some significant, well-documented changes to the [latex]\ce{_6^{14}C:_6^{12}C}[/latex] ratio. The accuracy of a straightforward application of this technique depends on the [latex]\ce{_6^{14}C:_6^{12}C}[/latex] ratio in a living plant being the same now as it was in an earlier era, but this is not always valid. Due to the increasing accumulation of [latex]\ce{CO2}[/latex] molecules (largely [latex]\ce{_6^{12}CO2}[/latex]) in the atmosphere caused by combustion of fossil fuels (in which essentially all of the [latex]\ce{_6^{14}C}[/latex] has decayed), the ratio of [latex]\ce{_6^{14}C:_6^{12}C}[/latex] in the atmosphere may be changing. This manmade increase in [latex]\ce{_6^{12}CO2}[/latex] in the atmosphere causes the [latex]\ce{_6^{14}C:_6^{12}C}[/latex] ratio to decrease, and this in turn affects the ratio in currently living organisms on the earth. Fortunately, however, we can use other data, such as tree dating via examination of annual growth rings, to calculate correction factors. With these correction factors, accurate dates can be determined. In general, radioactive dating only works for about 10 half-lives; therefore, the limit for carbon-14 dating is about 57,000 years.

Radioactive Dating Using Nuclides Other than Carbon-14

Radioactive dating can also use other radioactive nuclides with longer half-lives to date older events. For example, uranium-238 (which decays in a series of steps into lead-206) can be used for establishing the age of rocks (and the approximate age of the oldest rocks on earth). Since [latex]\ce{U{-}238}[/latex] has a half-life of 4.5 billion years, it takes that amount of time for half of the original [latex]\ce{U{-}238}[/latex] to decay into [latex]\ce{Pb{-}206}[/latex]. In a sample of rock that does not contain appreciable amounts of [latex]\ce{Pb{-}208}[/latex], the most abundant isotope of lead, we can assume that lead was not present when the rock was formed. Therefore, by measuring and analyzing the ratio of [latex]\ce{U{-}238:Pb{-}206}[/latex], we can determine the age of the rock. This assumes that all of the lead-206 present came from the decay of uranium-238. If there is additional lead-206 present, which is indicated by the presence of other lead isotopes in the sample, it is necessary to make an adjustment. Potassium-argon dating uses a similar method. [latex]\ce{K{-}40}[/latex] decays by positron emission and electron capture to form [latex]\ce{Ar{-}40}[/latex] with a half-life of 1.25 billion years. If a rock sample is crushed and the amount of [latex]\ce{Ar{-}40}[/latex] gas that escapes is measured, determination of the [latex]\ce{Ar{-}40:K{-}40}[/latex] ratio yields the age of the rock. Other methods, such as rubidium-strontium dating ([latex]\ce{Rb{-}87}[/latex] decays into [latex]\ce{Sr{-}87}[/latex] with a half-life of 48.8 billion years), operate on the same principle. To estimate the lower limit for the earth’s age, scientists determine the age of various rocks and minerals, making the assumption that the earth is older than the oldest rocks and minerals in its crust. As of 2014, the oldest known rocks on earth are the Jack Hills zircons from Australia, found by uranium-lead dating to be almost 4.4 billion years old.

Example 19.3.3: Radioactive Dating of Rocks

An igneous rock contains 9.58 [latex]\times[/latex] 10–5 g of [latex]\ce{U{-}238}[/latex] and 2.51 [latex]\times[/latex] 10–5 g of [latex]\ce{Pb{-}206}[/latex], and much, much smaller amounts of [latex]\ce{Pb{-}208}[/latex]. Determine the approximate time at which the rock formed.
[reveal-answer q=”626915″]Show Solution[/reveal-answer]
[hidden-answer a=”626915″]

The sample of rock contains very little [latex]\ce{Pb{-}208}[/latex], the most common isotope of lead, so we can safely assume that all the [latex]\ce{Pb{-}206}[/latex] in the rock was produced by the radioactive decay of [latex]\ce{U{-}238}[/latex]. When the rock formed, it contained all of the [latex]\ce{U{-}238}[/latex] currently in it, plus some [latex]\ce{U{-}238}[/latex] that has since undergone radioactive decay.

The amount of [latex]\ce{U{-}238}[/latex] currently in the rock is:

[latex]9.58\times {10}^{-5}\cancel{\text{g } \ce{U}}\times \left(\dfrac{\text{1 mol } \ce{U}}{238\cancel{\text{g } \ce{U}}}\right)=4.03\times {10}^{-7}\text{mol } \ce{U}[/latex]

Because when one mole of [latex]\ce{U{-}238}[/latex] decays, it produces one mole of [latex]\ce{Pb{-}206}[/latex], the amount of [latex]\ce{U{-}238}[/latex] that has undergone radioactive decay since the rock was formed is:

[latex]2.51\times {10}^{-5}\cancel{\text{g } \ce{Pb}}\times \left(\dfrac{1\cancel{\text{mol } \ce{Pb}}}{206\cancel{\text{g } \ce{Pb}}}\right)\times \left(\dfrac{\text{1 mol } \ce{U}}{1\cancel{\text{mol } \ce{Pb}}}\right)=1.22\times {10}^{-7}\text{mol } \ce{U}[/latex]

The total amount of [latex]\ce{U{-}238}[/latex] originally present in the rock is therefore:

[latex]4.03\times {10}^{-7}\text{mol}+1.22\times {10}^{-7}\text{mol}=5.25\times {10}^{-7}\text{mol } \ce{U}[/latex]

The amount of time that has passed since the formation of the rock is given by:

[latex]t=-\dfrac{1}{\lambda }\text{ln}\left(\dfrac{{N}_{t}}{{N}_{0}}\right)[/latex]

with N0 representing the original amount of [latex]\ce{U{-}238}[/latex] and Nt representing the present amount of [latex]\ce{U{-}238}[/latex].

[latex]\ce{U{-}238}[/latex] decays into [latex]\ce{Pb{-}206}[/latex] with a half-life of 4.5 [latex]\times[/latex] 109 y, so the decay constant λ is:

[latex]\lambda =\dfrac{\text{ln 2}}{{t}_{1\text{/}2}}=\dfrac{0.693}{4.5\times {10}^{9}\text{y}}=1.54\times {10}^{-10}{\text{y}}^{-1}[/latex]

Substituting and solving, we have:

[latex]t=-\dfrac{1}{1.54\times {10}^{-10}{\text{y}}^{-1}}\text{ln}\left(\dfrac{4.03\times {10}^{-7}\cancel{\text{mol U}}}{5.25\times {10}^{-7}\cancel{\text{mol U}}}\right)=1.7\times {10}^{9}\text{y}[/latex]

Therefore, the rock is approximately 1.7 billion years old.

[/hidden-answer]

Check Your Learning

Key Concepts and Summary

Nuclei that have unstable n:p ratios undergo spontaneous radioactive decay. The most common types of radioactivity are α decay, β decay, γ emission, positron emission, and electron capture. Nuclear reactions also often involve γ rays, and some nuclei decay by electron capture. Each of these modes of decay leads to the formation of a new nucleus with a more stable n:p ratio. Some substances undergo radioactive decay series, proceeding through multiple decays before ending in a stable isotope. All nuclear decay processes follow first-order kinetics, and each radioisotope has its own characteristic half-life, the time that is required for half of its atoms to decay. Because of the large differences in stability among nuclides, there is a very wide range of half-lives of radioactive substances. Many of these substances have found useful applications in medical diagnosis and treatment, determining the age of archaeological and geological objects, and more.

Key Equations

  • [latex]\text{decay rate}= \lambda{N}[/latex]
  • [latex]{t}_{1\text{/}2}=\dfrac{\text{ln 2}}{\lambda }=\dfrac{0.693}{\lambda }[/latex]

Try It

  1. What are the types of radiation emitted by the nuclei of radioactive elements?
  3. What is the change in the nucleus that results from the following decay scenarios?
    1. emission of a β particle
    2. emission of a β+ particle
    3. capture of an electron
  5. Why is electron capture accompanied by the emission of an X-ray?
  7. Which of the following nuclei is most likely to decay by positron emission? Explain your choice.
    1. chromium-53
    2. manganese-51
    3. iron-59
  9. The following nuclei do not lie in the band of stability. How would they be expected to decay?
    1. [latex]\ce{_{15}^{28}P}[/latex]
    2. [latex]\ce{_{92}^{235}U}[/latex]
    3. [latex]\ce{_{20}^{37}Ca}[/latex]
    4. [latex]\ce{_3^9Li}[/latex]
    5. [latex]\ce{_{96}^{245}Cm}[/latex]

[reveal-answer q=”725278″]Show Solution[/reveal-answer]
[hidden-answer a=”725278″]

  1. α (helium nuclei), β (electrons), β+ (positrons), and η (neutrons) may be emitted from a radioactive element, all of which are particles; γ rays also may be emitted.
  2. The changes are as follows:
    1. conversion of a neutron to a proton: [latex]{}_{0}^{1}\text{n}\longrightarrow {}_{1}^{1}\text{p}+{}_{+1}^{0}\text{e}[/latex]
    2. conversion of a proton to a neutron; the positron has the same mass as an electron and the same magnitude of positive charge as the electron has negative charge; when the n:p ratio of a nucleus is too low, a proton is converted into a neutron with the emission of a positron: [latex]{}_{1}^{1}\text{p}\longrightarrow {}_{0}^{1}\text{n}+{}_{+1}^{0}\text{e}[/latex]
    3. In a proton-rich nucleus, an inner atomic electron can be absorbed. In simplifies form, this changes a proton into a neutron: [latex]{}_{1}^{1}\text{p}+{}_{-1}^{0}\text{e}\longrightarrow {}_{0}^{1}\text{p}[/latex]
  3. The electron pulled into the nucleus was most likely found in the 1s orbital. As an electron falls from a higher energy level to replace it, the difference in the energy of the replacement electron in its two energy levels is given off as an X-ray.
  4. Manganese-51 is most likely to decay by positron emission. The n:p ratio for Cr-53 is [latex]\frac{29}{24}[/latex] = 1.21; for [latex]\ce{Mn{-}51}[/latex], it is [latex]\frac{26}{25}[/latex] = 1.04; for [latex]\ce{Fe{-}59}[/latex], it is [latex]\frac{33}{26}[/latex] = 1.27. Positron decay occurs when the n:p ratio is low. [latex]\ce{Mn{-}51}[/latex] has the lowest n:p ration and therefore is most likely to decay by positron emission. Besides, [latex]\ce{_{24}^{53}Cr}[/latex] is a stable isotope, and [latex]\ce{_{26}^{59}Fe}[/latex] decays by beta emission.
  5. The expected decay for each nucleus is as follows:
    1. too many neutrons, β decay
    2. atomic number greater than 82, α decay
    3. too few neutrons, positron emission
    4. too many neutrons, β decay
    5. atomic number greater than 83, α decay
  6. The reactions are as follows:
    • [latex]\ce{_{92}^{238}U}\longrightarrow \ce{_{90}^{234}Th}+\ce{_2^4He}[/latex]
    • [latex]\ce{_{90}^{234}Th}\longrightarrow \ce{_{91}^{234}Pa}+{}_{-1}^{0}\text{e}[/latex]
    • [latex]\ce{_{91}^{234}Pa}\longrightarrow \ce{_{92}^{234}U}+{}_{-1}^{0}\text{e}[/latex]
    • [latex]\ce{_{92}^{234}U}\longrightarrow \ce{_{90}^{230}Th}+\ce{_2^4He}[/latex]
    • [latex]\ce{_{90}^{230}Th}\longrightarrow \ce{_{88}^{226}Ra}+\ce{_2^4He}[/latex]
    • [latex]\ce{_{88}^{226}Ra}\longrightarrow \ce{_{86}^{222}Rn}+\ce{_2^4He}[/latex]
    • [latex]\ce{_{86}^{222}Rn}\longrightarrow \ce{_{84}^{218}Po}+\ce{_2^4He}[/latex]
  7. Half-life is the time required for half the atoms in a sample to decay. Example (answers may vary): For [latex]\ce{C{-}14}[/latex], the half-life is 5770 years. A 10-g sample of [latex]\ce{C{-}14}[/latex] would contain 5 g of [latex]\ce{C{-}14}[/latex] after 5770 years; a 0.20-g sample of [latex]\ce{C{-}14}[/latex]would contain 0.10 g after 5770 years.
  8. 1000 years is 0.04 half-lives. The fraction that remains after 0.04 half-lives is [latex]{\left(\frac{1}{2}\right)}^{0.04}=0.973[/latex] or 97.3%
  9. PV = nRT
    1. nRn = [latex]\frac{PV}{RT}=\frac{\left(\text{1 atm}\right)\left(0.0001\text{mL}\times \text{1 L/}{10}^{3}\text{mL}\right)}{\left(0.08206\text{L atm}{\text{mol}}^{-1}{\text{K}}^{-1}\right)\left(273.15\text{K}\right)}[/latex] = 4.4614 [latex]\times[/latex] 10–9 mol
    2. nRn produced = nRn decayed
    3. mass Ra lost = 4.4614 [latex]\times[/latex] 10–9 mol [latex]\times[/latex] [latex]\frac{\text{226 g}}{\text{mol}}[/latex] = 1.00827 [latex]\times[/latex] 10–6 g
    4. mass Ra remaining after 24 h = 1 – (1.00827 [latex]\times[/latex] 10–6 g) = 9.9999899 [latex]\times[/latex] 10–1 g
    5. ln [latex]\frac{{c}_{0}}{c}=\lambda t[/latex] = ln [latex]\frac{1.000}{9.9999899\times {10}^{-1}}=\lambda \left(\text{24 h}\right)[/latex] = 4.3785 [latex]\times[/latex] 10–7
    6. λ = 4.2015 [latex]\times[/latex] 10–8 h–1
    7. [latex]{t}_{1\text{/}2}=\frac{0.693}{\lambda }=\frac{0.693}{4.2015\times {10}^{-8}}[/latex] = 1.6494 [latex]\times[/latex] 107 h
    8. = 1.6494 [latex]\times[/latex] 107 h [latex]\times[/latex] [latex]\frac{\text{1 d}}{\text{24 h}}\times \frac{\text{1 y}}{\text{365 d}}[/latex] = 1.883 [latex]\times[/latex] 103 y or 2 [latex]\times[/latex] 103 y
  10. (Recall that radioactive decay is a first-order process.)
    1. λ = [latex]\frac{0.693}{{t}_{\frac{1}{2}}}=\frac{0.693}{6.0\text{h}}[/latex] = 0.12 h–1
  11. The c are as follows:
    1. [latex]\ce{_{37}^{87}Rb}\longrightarrow \ce{_{38}^{87}Sr}+{}_{-1}^{0}\text{e}[/latex]
    2. [latex]\ce{_{38}^{87}Sr}[/latex] is a stable isotope and does not decay further. Calculate the value of the decay rate constant for [latex]{}_{37}^{87}\text{Rb}[/latex], remembering that all radioactive decay is first order:
    3. [latex]\lambda =\frac{0.693}{4.7\times {10}^{10}\text{y}}=1.47\times {10}^{-11}{\text{y}}^{-1}[/latex]
    4. Calculate the number of moles of [latex]\ce{_{37}^{87}Rb}[/latex] and [latex]\ce{_{38}^{87}Sr}[/latex] found in the sample at time t:
    5. [latex]\begin{array}{l}8.23\text{mg}\times \frac{\text{1 g}}{\text{1000 mg}}\times \frac{\text{1 mol}}{87.0\text{g}}=9.46\times {10}^{-5}\text{mol of}\ce{_{37}^{87}Rb}\\ 0.47\text{mg}\times \frac{\text{1 g}}{\text{1000 mg}}\times \frac{\text{1 mol}}{87.0\text{g}}=5.40\times {10}^{-6}\text{mol of}{}_{38}^{87}\text{Sr}\end{array}[/latex]
    6. Each mol of [latex]\ce{_{37}^{87}Rb}[/latex] that disappeared (by radioactive decay of the [latex]\ce{_{37}^{87}Rb}[/latex] initially present in the rock) produced 1 mol of [latex]\ce{_{38}^{87}Sr}[/latex]. Hence the number of moles of [latex]\ce{_{38}^{87}Rb}[/latex] that disappeared by radioactive decay equals the number of moles of [latex]\ce{_{38}^{87}Sr}[/latex] that were produced. This amount consists of the 5.40 [latex]\times[/latex] 10–6 mol of [latex]\ce{_{38}^{87}Sr}[/latex] found in the rock at time t if all the [latex]\ce{_{38}^{87}Sr}[/latex] present at time t resulted from radioactive decay of [latex]\ce{_{37}^{87}Rb}[/latex] and no strontium-87 was present initially in the rock. Using this assumption, we can calculate the total number of moles of rubidium-87 initially present in the rock:
    7. Total number of moles of [latex]\ce{_{37}^{87}Rb}[/latex] initially present in the rock at time t 0 = number of moles of [latex]\ce{_{37}^{87}Rb}[/latex] at time t + number of moles of [latex]\ce{_{37}^{87}Rb}[/latex] that decayed during the time interval t – t0 = number of moles of [latex]\ce{_{37}^{87}Rb}[/latex] measured at time t + number of moles of [latex]\ce{_{38}^{87}Sr}[/latex] measured at time t = 9.46 [latex]\times[/latex] 10–5 mol + 5.40 [latex]\times[/latex] 10–6 mol = 1.00 [latex]\times[/latex] 10–4 mol
    8. The number of moles can be substituted for concentrations in the expression:
    9. [latex]\text{ln}\frac{{c}_{0}}{{c}_{t}}=\lambda t[/latex]
    10. Thus:
    11. [latex]\begin{array}{l}\text{ln}\frac{1.00\times {10}^{-4}\text{mol}}{9.46\times {10}^{-5}\text{mol}}=\left(1.47\times {10}^{-11}\right)t\\ t=\left(\mathrm{ln}\frac{1.00\times {10}^{-4}}{9.46\times {10}^{-5}}\right)\left(\frac{1}{1.47\times {10}^{-11}{\text{y}}^{-1}}\right)\end{array}[/latex]
    12. = 3.8 [latex]\times[/latex] 109 y = 3.8 billion years = age of the rock sample
    13. The rock would be younger than the age calculated in part (a). If [latex]\ce{Sr}[/latex] was originally in the rock, the amount produced by radioactive decay would equal the present amount minus the initial amount. As this amount would be smaller than the amount used to calculate the age of the rock and the age is proportional to the amount of [latex]\ce{Sr}[/latex], the rock would be younger.
  12. [latex]\ce{_{94}^{239}Pu}[/latex] has a half-life of 2.411 [latex]\times[/latex] 104 y. Calculate the value of λ and then determine the amount of plutonium-239 remaining after 4.7 [latex]\times[/latex] 109 y:
    1. λt = λ(2.411 [latex]\times[/latex] 104 y) = ln [latex]\left(\frac{1.0000}{0.5000}\right)[/latex] = 0.6931
    2. λ = [latex]\frac{0.6931}{2.411}[/latex] [latex]\times[/latex] 104 y = 2.875 [latex]\times[/latex] 10–5 y–1
    3. Then:
    4. ln [latex]\frac{{c}_{0}}{c}[/latex] = λt
    5. ln [latex]\left(\frac{1.000}{c}\right)[/latex] = 2.875 [latex]\times[/latex] 10–5 y–1 [latex]\times[/latex] 4.7 [latex]\times[/latex] 109 y
    6. ln c = –1.351 [latex]\times[/latex] 105
    7. c = 0
    8. This calculation shows that no [latex]\ce{Pu{-}239}[/latex] could remain since the formation of the earth. Consequently, the plutonium now present could not have been formed with the uranium.
  13. Find the mass difference of the starting mass and the total masses of the final products. Then use the conversion for mass to energy to find the energy released:
    1. 8.0246 – 8.0053 – 0.00055 = 0.01875 amu
    2. 0.01875 amu [latex]\times[/latex] 1.6605 [latex]\times[/latex] 10–27 kg/amu = 3.113 [latex]\times[/latex] 10–29 kg
    3. E = mc2 = (3.113 [latex]\times[/latex] 10–29 kg)(2.9979 [latex]\times[/latex] 108 m/s)2
    4. = 2.798 [latex]\times[/latex] 10–12 kg m2/s2 = 2.798 [latex]\times[/latex] 10–12 J/nucleus
    5. 2.798 [latex]\times[/latex] 10–12 J/nucleus [latex]\times[/latex] [latex]\frac{\text{1 MeV}}{1.602177\times {10}^{-13}\text{J}}[/latex] = 17.5 MeV
  14. The balanced equations are as follows:
    1. [latex]\ce{_{83}^{212}Bi}\longrightarrow \ce{_{84}^{212}Po}+{}_{-1}^{0}\text{e}[/latex]
    2. [latex]\ce{_5^8B}\longrightarrow {}_{4}^{8}\text{B}\text{e}+{}_{-1}^{0}\text{e}[/latex]
    3. [latex]{}_{92}^{238}\text{U}+{}_{0}^{1}\text{n}\longrightarrow \ce{_{93}^{239}Np}+\ce{_{-1}^{0}Np}[/latex], [latex]\ce{_{93}^{239}Np}\longrightarrow \ce{_{94}^{239}Pu}+{}_{-1}^{0}\text{e}[/latex]
    4. [latex]\ce{_{38}^{90}Sr}\longrightarrow {}_{39}^{90}\text{Y}+{}_{-1}^{0}\text{e}[/latex]

[/hidden-answer]

Glossary

alpha (α) decay: loss of an alpha particle during radioactive decay

beta (β) decay: breakdown of a neutron into a proton, which remains in the nucleus, and an electron, which is emitted as a beta particle

daughter nuclide: nuclide produced by the radioactive decay of another nuclide; may be stable or may decay further

electron capture: combination of a core electron with a proton to yield a neutron within the nucleus

gamma (γ) emission: decay of an excited-state nuclide accompanied by emission of a gamma ray

half-life (t1/2): time required for half of the atoms in a radioactive sample to decay

parent nuclide: unstable nuclide that changes spontaneously into another (daughter) nuclide

positron emission: (also, β+ decay) conversion of a proton into a neutron, which remains in the nucleus, and a positron, which is emitted

radioactive decay: spontaneous decay of an unstable nuclide into another nuclide

radioactive decay series: chains of successive disintegrations (radioactive decays) that ultimately lead to a stable end-product

radiocarbon dating: highly accurate means of dating objects 30,000–50,000 years old that were derived from once-living matter; achieved by calculating the ratio of [latex]\ce{_6^{14}C:6}^{12}C}[/latex] in the object vs. the ratio of [latex]\ce{_6^{14}C:_6^{12}C}[/latex] in the present-day atmosphere

radiometric dating: use of radioisotopes and their properties to date the formation of objects such as archeological artifacts, formerly living organisms, or geological formations

Licenses and Attributions (Click to expand)

CC licensed content, Shared previously

All rights reserved content

  • How to Build a Cloud Chamber!. Authored by: Jefferson Lab. Located at: https://youtu.be/pewTySxfTQk. License: Other. License Terms: Standard YouTube License
  1. The “m” in Tc-99m stands for “metastable,” indicating that this is an unstable, high-energy state of Tc-99. Metastable isotopes emit γ radiation to rid themselves of excess energy and become (more) stable. ↵
definition

License

Icon for the Creative Commons Attribution-NonCommercial-ShareAlike 4.0 International License

Chemistry Fundamentals Copyright © by Dr. Julie Donnelly, Dr. Nicole Lapeyrouse, and Dr. Matthew Rex is licensed under a Creative Commons Attribution-NonCommercial-ShareAlike 4.0 International License, except where otherwise noted.

Share This Book