3.6 The Shape of Atomic Orbitals – Chemistry LibreTexts

https://chem.libretexts.org/Bookshelves/General_Chemistry/Map%3A_A_Molecular_Approach_(Tro)/07%3A_The_Quantum-Mechanical_Model_of_the_Atom/7.06%3A_The_Shape_of_Atomic_Orbitals

An orbital is the quantum mechanical refinement of Bohr’s orbit. In contrast to his concept of a simple circular orbit with a fixed radius, orbitals are mathematically derived regions of space with different probabilities of containing an electron.

One way of representing electron probability distributions was illustrated previously for the orbital of hydrogen. Because [latex]\Psi^2[/latex] gives the probability of finding an electron in a given volume of space (such as a cubic picometer), a plot of [latex]\Psi^2[/latex] versus distance from the nucleus (r) is a plot of the probability density. The 1s orbital is spherically symmetrical, so the probability of finding a 1s electron at any given point depends only on its distance from the nucleus. The probability density is greatest at r = 0 (at the nucleus) and decreases steadily with increasing distance. At very large values of r, the electron probability density is very small but not zero.

In contrast, we can calculate the radial probability (the probability of finding a 1s electron at a distance r from the nucleus) by adding together the probabilities of an electron being at all points on a series of x spherical shells of radius r₁, r₂, r₃,…, r_x-1, r_x. In effect, we are dividing the atom into very thin concentric shells, much like the layers of an onion (Figure 3.6.1a), and calculating the probability of finding an electron on each spherical shell. Recall that the electron probability density is greatest at r = 0 (Figure 3.6.1b), so the density of dots is greatest for the smallest spherical shells in part (a) in Figure 3.6.1. In contrast, the surface area of each spherical shell is equal to 4πr², which increases very rapidly with increasing r (Figure 3.6.1c). Because the surface area of the spherical shells increases more rapidly with increasing r than the electron probability density decreases, the plot of radial probability has a maximum at a particular distance (Figure 3.6.1d). Most important, when r is very small, the surface area of a spherical shell is so small that the total probability of finding an electron close to the nucleus is very low; at the nucleus, the electron probability vanishes (Figure 3.6.1d).

For the hydrogen atom, the peak in the radial probability plot occurs at r = 0.529 Å (52.9 pm), which is exactly the radius calculated by Bohr for the n = 1 orbit. Thus the most probable radius obtained from quantum mechanics is identical to the radius calculated by classical mechanics. In Bohr’s model, however, the electron was assumed to be at this distance 100% of the time, whereas in the Schrödinger model, it is at this distance only some of the time. The difference between the two models is attributable to the wavelike behavior of the electron and the Heisenberg uncertainty principle.

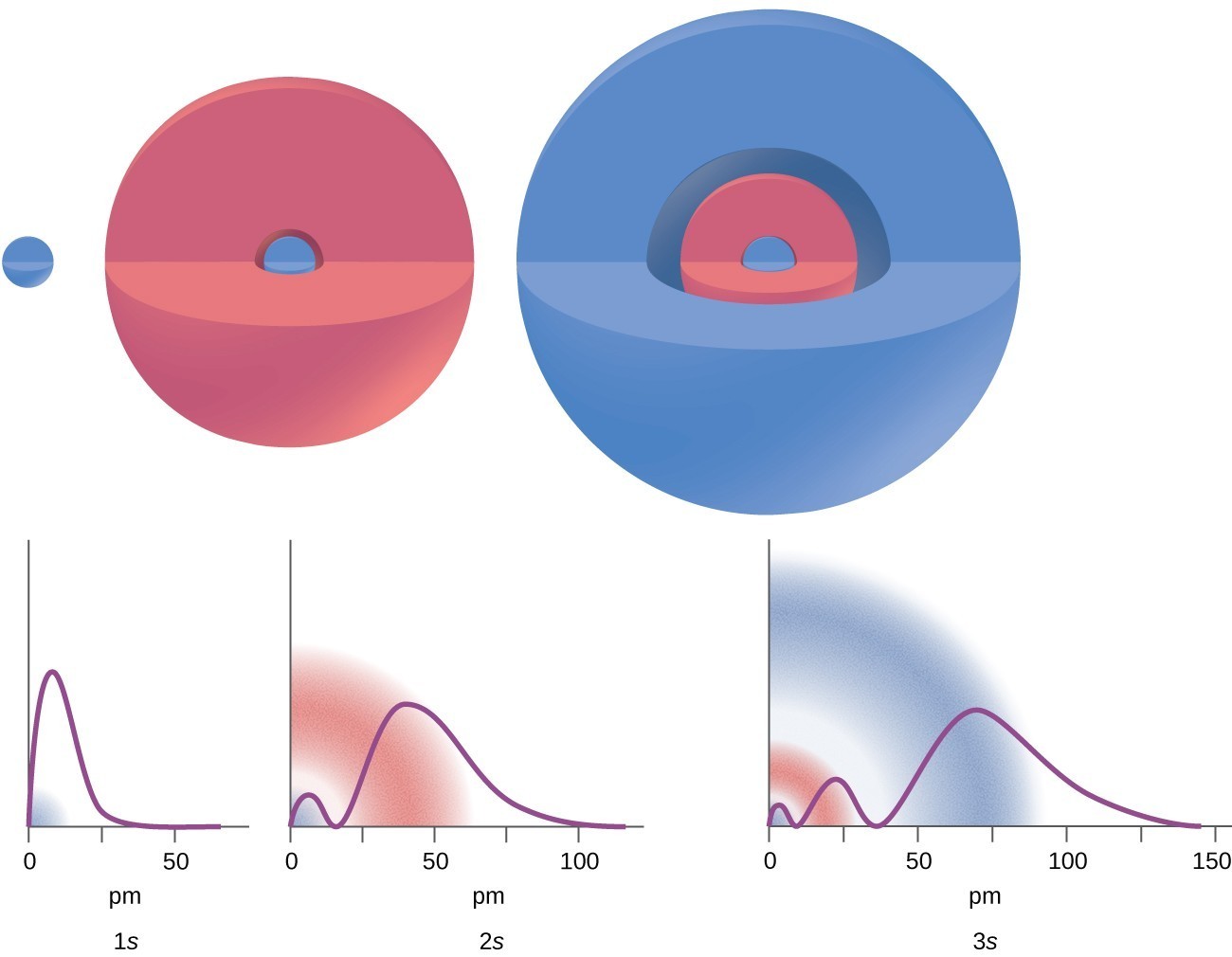
Figure 3.6.2 compares the electron probability densities for the hydrogen 1s, 2s, and 3s orbitals. Note that all three are spherically symmetrical. For the 2s and 3s orbitals, however (and for all other s orbitals as well), the electron probability density does not fall off smoothly with increasing r. Instead, a series of minima and maxima are observed in the radial probability plots (Figure 3.6.2c). The minima correspond to spherical nodes (regions of zero electron probability), which alternate with spherical regions of nonzero electron probability. The existence of these nodes is a consequence of changes of wave phase in the wavefunction [latex]\Psi[/latex].

s Orbitals (l = 0)

Three things happen to s orbitals as n increases (Figure 3.6.2):

1. They become larger, extending farther from the nucleus.
2. They contain more nodes. This is similar to a standing wave that has regions of significant amplitude separated by nodes, points with zero amplitude.
3. For a given atom, the s orbitals also become higher in energy as n increases because of their increased distance from the nucleus.

Orbitals are generally drawn as three-dimensional surfaces that enclose 90% of the electron density, as was shown for the hydrogen 1s, 2s, and 3s orbitals in part (b) in Figure 3.6.2. Although such drawings show the relative sizes of the orbitals, they do not normally show the spherical nodes in the 2s and 3s orbitals because the spherical nodes lie inside the 90% surface. Fortunately, the positions of the spherical nodes are not important for chemical bonding.

p Orbitals (l = 1)

Only s orbitals are spherically symmetrical. As the value of l increases, the number of orbitals in a given subshell increases, and the shapes of the orbitals become more complex. Because the 2p subshell has l = 1, with three values of m_l (-1, 0, and +1), there are three 2p orbitals.

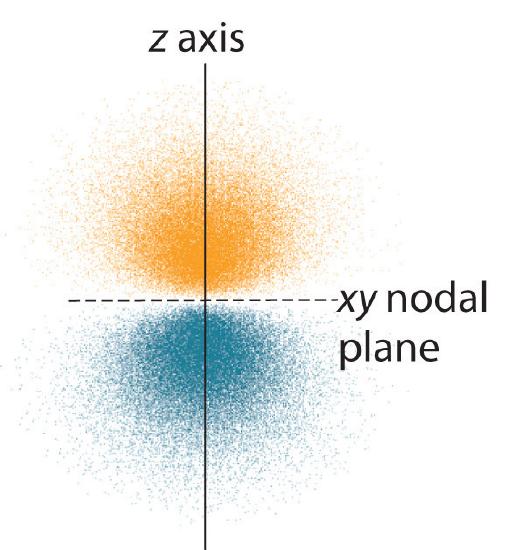
The electron probability distribution for one of the hydrogen 2p orbitals is shown in Figure 3.6.4. Because this orbital has two lobes of electron density arranged along the z axis, with an electron density of zero in the xy plane (i.e., the xy plane is a nodal plane), it is a 2p_z orbital. As shown in Figure 3.6.5, the other two 2p orbitals have identical shapes, but they lie along the x axis (2p_x) and y axis (2p_y), respectively. Note that each p orbital has just one nodal plane. In each case, the phase of the wave function for each of the 2p orbitals is positive for the lobe that points along the positive axis and negative for the lobe that points along the negative axis. It is important to emphasize that these signs correspond to the phase of the wave that describes the electron motion, not to positive or negative charges.

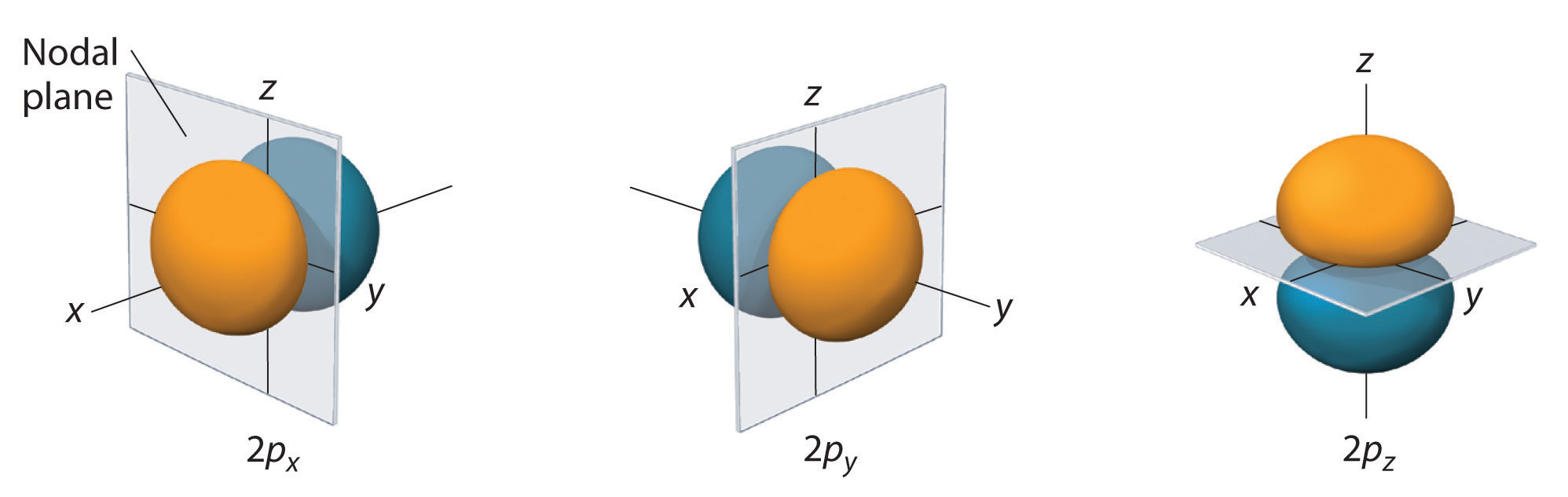
The surfaces shown enclose 90% of the total electron probability for the 2p_x, 2p_y, and 2p_z orbitals. Each orbital is oriented along the axis indicated by the subscript and a nodal plane that is perpendicular to that axis bisects each 2p orbital. The phase of the wave function is positive (orange) in the region of space where x, y, or z is positive and negative (blue) where x, y, or z is negative. Just as with the s orbitals, the size and complexity of the p orbitals for any atom increase as the principal quantum number n increases. The shapes of the 90% probability surfaces of the 3p, 4p, and higher-energy p orbitals are, however, essentially the same as those shown in Figure 3.6.5.

d Orbitals (l = 2)

Subshells with l = 2 have five d orbitals; the first principal shell to have a d subshell corresponds to n = 3. The five d orbitals have m_l values of -2, -1, 0, +1, and +2.

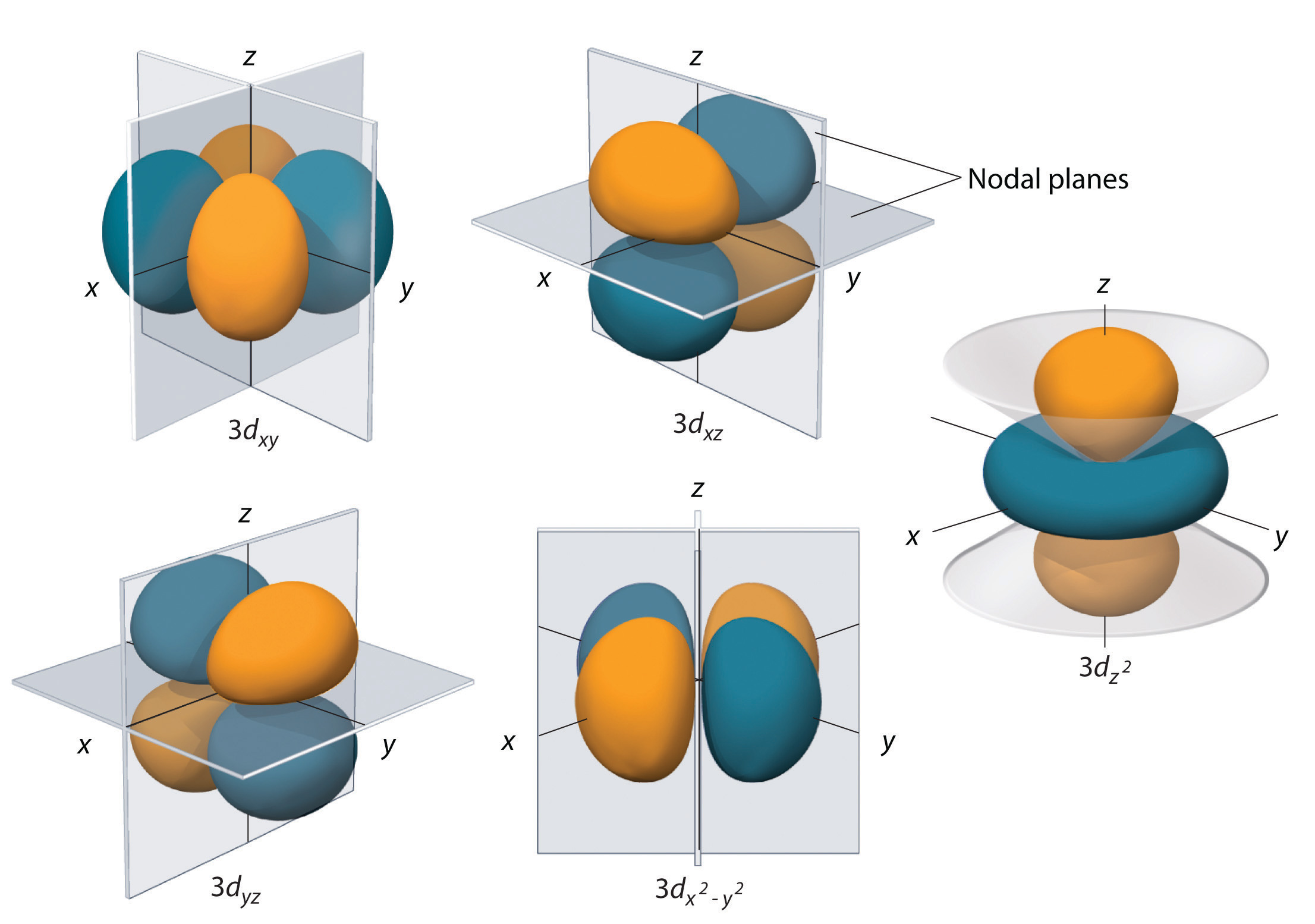
The hydrogen 3d orbitals, shown in Figure 3.6.6, have more complex shapes than the 2p orbitals. All five 3d orbitals contain two nodal surfaces, as compared to one for each p orbital and zero for each s orbital. In three of the d orbitals, the lobes of electron density are oriented between the x and y, x and x, and y and z planes; these orbitals are referred to as the 3d_xy, 3d_xz, and 3d_yz orbitals, respectively. A fourth d orbital has lobes lying along the x and y axes; this is the 3d_z^2–2 orbital. The fifth 3d orbital, called the 3d_z² orbital, has a unique shape: it looks like a 2p_z orbital combined with an additional doughnut of electron probability lying in the xy plane. Despite its peculiar shape, the 3d_z² orbital is mathematically equivalent to the other four and has the same energy. In contrast to p orbitals, the phase of the wave function for d orbitals is the same for opposite pairs of lobes. As shown in Figure 3.6.6, the phase of the wave function is positive for the two lobes of the dz² orbital that lie along the z axis, whereas the phase of the wave function is negative for the doughnut of electron density in the xy plane. Like the s and p orbitals, as n increases, the size of the d orbitals increases, but the overall shapes remain similar to those depicted in Figure 3.6.6.

f Orbitals (l = 3)

Principal shells with n = 4 can have subshells with l = 3 and m_l values of -3, -2, -1, 0, +1, +2, and +3. These subshells consist of seven f orbitals. Each f orbital has three nodal surfaces, so their shapes are complex. Because f orbitals are not particularly important for our purposes, we do not discuss them further, and orbitals with higher values of l are not discussed at all.

Orbital Energies

Although we have discussed the shapes of orbitals, we have said little about their comparative energies. We begin our discussion of orbital energies by considering atoms or ions with only a single electron (such as [latex]\ce{H}[/latex] or [latex]\ce{He^+}[/latex]).

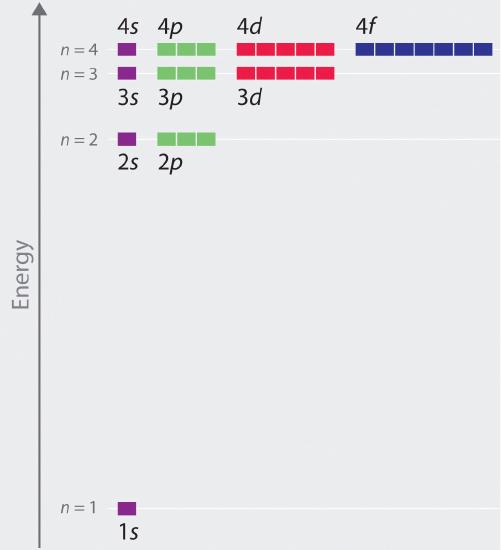
The relative energies of the atomic orbitals with n ≤ 4 for a hydrogen atom are plotted in Figure 3.6.8; note that the orbital energies depend on only the principal quantum number n. Consequently, the energies of the 2s and 2p orbitals of hydrogen are the same; the energies of the 3s, 3p, and 3d orbitals are the same; and so forth. Quantum mechanics predicts that in the hydrogen atom, all orbitals with the same value of n (e.g., the three 2p orbitals) are degenerate, meaning that they have the same energy. The orbital energies obtained for hydrogen using quantum mechanics are exactly the same as the allowed energies calculated by Bohr. In contrast to Bohr’s model, however, which allowed only one orbit for each energy level, quantum mechanics predicts that there are 4 orbitals with different electron density distributions in the n = 2 principal shell (one 2s and three 2p orbitals), 9 in the n = 3 principal shell, and 16 in the n = 4 principal shell.The different values of l and m_l for the individual orbitals within a given principal shell are not important for understanding the emission or absorption spectra of the hydrogen atom under most conditions, but they do explain the splittings of the main lines that are observed when hydrogen atoms are placed in a magnetic field. Figure 3.6.8 shows that the energy levels become closer and closer together as the value of n increases, as expected because of the [latex]\frac{1}{n^2}[/latex] dependence of orbital energies.

The energies of the orbitals (n) in any species with only one electron can be calculated by a minor variation of Bohr’s equation, which can be extended to other single-electron species by incorporating the nuclear charge Z (the number of protons in the nucleus), Rydberg constant (R) 1.097 × 10⁷ m^-1, Planck’s constant (h) 6.626 × 10^-34 joule seconds (J s), and the speed of light (c) 2.998 × 10⁸ m/s:

[latex]E=−\dfrac{Z^2}{n^2}Rhc \label{6.6.1}[/latex]

In general, both energy and radius decrease as the nuclear charge increases. Thus the most stable orbitals (those with the lowest energy) are those closest to the nucleus. For example, in the ground state of the hydrogen atom, the single electron is in the 1s orbital, whereas in the first excited state, the atom has absorbed energy and the electron has been promoted to one of the n = 2 orbitals. In ions with only a single electron, the energy of a given orbital depends on only n, and all subshells within a principal shell, such as the p_x, p_y, and p_z orbitals, are degenerate.

Glossary

Degenerate orbitals: orbitals that have the same energy

Nodes: regions of zero electron probability

Probability density: the probability of finding an electron in a given volume of space (such as a cubic picometer), a plot of [latex]\Psi^2[/latex] versus distance from the nucleus (r)

Radial probability: the probability of finding a 1s electron at a distance r from the nucleus

Contributors and Attributions